Since electrical signals are strongly attenuated in ionic solutions, where the Debye length is typically of the order of 10nm or less for mM range salt concentrations, Faradaic reactions are required to communicate via low frequency signals for lablets as well as for charging purposes. Modelling of these strongly nonlinear phenomena at digital voltages (0-3V) is complex in low ionic strength solutions, where space charge formation through salt depletion can occur, and literature integration of these nonlinear effects together with reactions is limited. We showed how to couple such electrochemical models with electrical circuits modelling lablets and set up a symbolic algebra program to generate and numerically solve the nonlinearly coupled differential equations arising.
Typical simulations are shown in fig. 1. Over short distances of ca. 1 µm, direct electrical communication is feasible in weak electrolyte solutions, but for longer distances communication via slower Faradaic reaction response is preferable. This is possible using the bipolar field effect created by a sustained Faradaic reaction, and this may be used for charging too. Charging a diode through solution has been modelled and experimentally studied with MnO2-Fe3O4 and MnO2-PPy supercapacitors. A longer range of electrical communication (up to 100µm) can be potentially achieved making use of electrochemically produced redox transmitters to transmit information via diffusion with concentration signals then converted back into Nernstian voltages at the receiver.

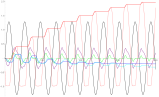
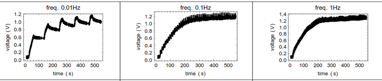

Figure 1 Charging and communication with lablets. Top Left: Equivalent circuit with nonlinear solution capacitor components (leakage current ignored). Top right: simulation of various potentials including the staircase potential across the diode. Middle experimental charging of cm-scale MnO2-Ppy supercap at three different frequencies up to 1.4V. The reversions arise from leakage current which can be estimated by this approach iL =deltaV/2RESR. Bottom Left: concentration v/s time of chemical field at different distances from the source lablet for a defined sequence of voltages (shown in black). The initial concentration of chemical was 25mM and applied voltage was 1.5V. As we move away from the source lablet, patterns in the chemical field fades, hence restricting the communication to work in finite distances from the lablet. Bottom Right: analog voltages the receiver lablet senses based on the concentration field created by the source lablet at different distances v/s time. The voltages are calculated from Nernst equation assuming the equilibrium value as 0.4 V.